The inverse hyperbolic tangent tanh^(-1)z (Zwillinger 1995, p. 481; Beyer 1987, p. 181), sometimes called the area hyperbolic tangent (Harris and Stocker 1998, p. 267), is the multivalued function that is the inverse function of the hyperbolic tangent.

Show Mobile NoticeShow All NotesHide All Notes

Section 3-7 : Derivatives of Inverse Trig Functions

Calculate the derivative of the function (y = arccos x arctan x ) at (x = 0. ) Example 9 Using the chain rule, derive the formula for the derivative of the inverse sine function. Find the Derivative - d/dx y=arctan(1/x) Differentiate using the chain rule, which states that is where. Tap for more steps. To apply the Chain Rule, set as. The derivative of arctan returns an algebraic expression. We can use this expression to find the derivative of inverse trigonometric functions. In our discussion, we’ll understand how to differentiate arctan and use the new derivative rule to differentiate more complex functions. Make sure to have your notes handy!

In this section we are going to look at the derivatives of the inverse trig functions. In order to derive the derivatives of inverse trig functions we’ll need the formula from the last section relating the derivatives of inverse functions. If (fleft( x right)) and (gleft( x right)) are inverse functions then,

[g'left( x right) = frac{1}{{f'left( {gleft( x right)} right)}}]

Recall as well that two functions are inverses if (fleft( {gleft( x right)} right) = x) and (gleft( {fleft( x right)} right) = x).

We’ll go through inverse sine, inverse cosine and inverse tangent in detail here and leave the other three to you to derive if you’d like to.

Inverse Sine

Let’s start with inverse sine. Here is the definition of the inverse sine.

[y = {sin ^{ - 1}}xhspace{0.5in} Leftrightarrow hspace{0.5in}sin y = xhspace{0.25in}{mbox{for}}, - frac{pi }{2} le y le frac{pi }{2}]

So, evaluating an inverse trig function is the same as asking what angle (i.e. (y)) did we plug into the sine function to get (x). The restrictions on (y) given above are there to make sure that we get a consistent answer out of the inverse sine. We know that there are in fact an infinite number of angles that will work and we want a consistent value when we work with inverse sine. Using the range of angles above gives all possible values of the sine function exactly once. If you’re not sure of that sketch out a unit circle and you’ll see that that range of angles (the (y)’s) will cover all possible values of sine.

Note as well that since ( - 1 le sin left( y right) le 1) we also have ( - 1 le x le 1).

Let’s work a quick example.

Example 1 Evaluate (displaystyle {sin ^{ - 1}}left( {frac{1}{2}} right)) Show Solution

So, we are really asking what angle (y) solves the following equation.

[sin left( y right) = frac{1}{2}]

and we are restricted to the values of (y) above.

From a unit circle we can quickly see that (y = frac{pi }{6}).

We have the following relationship between the inverse sine function and the sine function.

[sin left( {{{sin }^{ - 1}}x} right) = xhspace{0.5in}{sin ^{ - 1}}left( {sin x} right) = x]

In other words they are inverses of each other. This means that we can use the fact above to find the derivative of inverse sine. Let’s start with,

[fleft( x right) = sin xhspace{0.5in}gleft( x right) = {sin ^{ - 1}}x]

Then,

[g'left( x right) = frac{1}{{f'left( {gleft( x right)} right)}} = frac{1}{{cos left( {{{sin }^{ - 1}}x} right)}}]

This is not a very useful formula. Let’s see if we can get a better formula. Let’s start by recalling the definition of the inverse sine function.

[y = {sin ^{ - 1}}left( x right)hspace{0.5in} Rightarrow hspace{0.5in}x = sin left( y right)]

Using the first part of this definition the denominator in the derivative becomes,

[cos left( {{{sin }^{ - 1}}x} right) = cos left( y right)]

Now, recall that

[{cos ^2}y + {sin ^2}y = 1hspace{0.5in} Rightarrow hspace{0.5in}cos y = sqrt {1 - {{sin }^2}y} ]

Using this, the denominator is now,

[cos left( {{{sin }^{ - 1}}x} right) = cos left( y right) = sqrt {1 - {{sin }^2}y} ]

Now, use the second part of the definition of the inverse sine function. The denominator is then,

[cos left( {{{sin }^{ - 1}}x} right) = sqrt {1 - {{sin }^2}y} = sqrt {1 - {x^2}} ]

Putting all of this together gives the following derivative.

[frac{d}{{dx}}left( {{{sin }^{ - 1}}x} right) = frac{1}{{sqrt {1 - {x^2}} }}]

Inverse Cosine

Now let’s take a look at the inverse cosine. Here is the definition for the inverse cosine.

[y = {cos ^{ - 1}}xhspace{0.5in} Leftrightarrow hspace{0.5in}cos y = xhspace{0.25in}{mbox{for}},0 le y le pi ]

As with the inverse sine we’ve got a restriction on the angles, (y), that we get out of the inverse cosine function. Again, if you’d like to verify this a quick sketch of a unit circle should convince you that this range will cover all possible values of cosine exactly once. Also, we also have ( - 1 le x le 1) because ( - 1 le cos left( y right) le 1).

Example 2 Evaluate (displaystyle {cos ^{ - 1}}left( { - frac{{sqrt 2 }}{2}} right)). Show Solution

As with the inverse sine we are really just asking the following.

[cos y = - frac{{sqrt 2 }}{2}]

where (y) must meet the requirements given above. From a unit circle we can see that we must have (y = frac{{3pi }}{4}).

The inverse cosine and cosine functions are also inverses of each other and so we have, [cos left( {{{cos }^{ - 1}}x} right) = xhspace{0.5in}{cos ^{ - 1}}left( {cos x} right) = x]

To find the derivative we’ll do the same kind of work that we did with the inverse sine above. If we start with

[fleft( x right) = cos xhspace{0.5in}gleft( x right) = {cos ^{ - 1}}x]

then,

[g'left( x right) = frac{1}{{f'left( {gleft( x right)} right)}} = frac{1}{{ - sin left( {{{cos }^{ - 1}}x} right)}}]

Simplifying the denominator here is almost identical to the work we did for the inverse sine and so isn’t shown here. Upon simplifying we get the following derivative.

[frac{d}{{dx}}left( {{{cos }^{ - 1}}x} right) = - frac{1}{{sqrt {1 - {x^2}} }}]

So, the derivative of the inverse cosine is nearly identical to the derivative of the inverse sine. The only difference is the negative sign.

Inverse Tangent

Here is the definition of the inverse tangent.

[y = {tan ^{ - 1}}xhspace{0.5in} Leftrightarrow hspace{0.5in}tan y = xhspace{0.25in}{mbox{for}}, - frac{pi }{2} < y < frac{pi }{2}]

Again, we have a restriction on (y), but notice that we can’t let (y) be either of the two endpoints in the restriction above since tangent isn’t even defined at those two points. To convince yourself that this range will cover all possible values of tangent do a quick sketch of the tangent function and we can see that in this range we do indeed cover all possible values of tangent. Also, in this case there are no restrictions on (x) because tangent can take on all possible values.

Example 3 Evaluate ({tan ^{ - 1}}1). Show Solution

Here we are asking,

[tan y = 1]

where (y) satisfies the restrictions given above. From a unit circle we can see that (y = frac{pi }{4}).

Because there is no restriction on (x) we can ask for the limits of the inverse tangent function as (x) goes to plus or minus infinity. To do this we’ll need the graph of the inverse tangent function. This is shown below.

From this graph we can see that

[mathop {lim }limits_{x to infty } {tan ^{ - 1}}x = frac{pi }{2}hspace{0.5in}hspace{0.25in}mathop {lim }limits_{x to - infty } {tan ^{ - 1}}x = - frac{pi }{2}]

The tangent and inverse tangent functions are inverse functions so,

[tan left( {{{tan }^{ - 1}}x} right) = xhspace{0.5in}{tan ^{ - 1}}left( {tan x} right) = x]

Therefore, to find the derivative of the inverse tangent function we can start with

[fleft( x right) = tan xhspace{0.5in}gleft( x right) = {tan ^{ - 1}}x]

We then have,

[g'left( x right) = frac{1}{{f'left( {gleft( x right)} right)}} = frac{1}{{{{sec }^2}left( {{{tan }^{ - 1}}x} right)}}]

Simplifying the denominator is similar to the inverse sine, but different enough to warrant showing the details. We’ll start with the definition of the inverse tangent.

[y = {tan ^{ - 1}}xhspace{0.5in} Rightarrow hspace{0.5in}tan y = x]

The denominator is then,

[{sec ^2}left( {{{tan }^{ - 1}}x} right) = {sec ^2}y]

Now, if we start with the fact that

[{cos ^2}y + {sin ^2}y = 1]

and divide every term by cos² (y) we will get,

[1 + {tan ^2}y = {sec ^2}y]

The denominator is then,

[{sec ^2}left( {{{tan }^{ - 1}}x} right) = {sec ^2}y = 1 + {tan ^2}y]

Finally using the second portion of the definition of the inverse tangent function gives us,

[{sec ^2}left( {{{tan }^{ - 1}}x} right) = 1 + {tan ^2}y = 1 + {x^2}]

The derivative of the inverse tangent is then,

[frac{d}{{dx}}left( {{{tan }^{ - 1}}x} right) = frac{1}{{1 + {x^2}}}]

There are three more inverse trig functions but the three shown here the most common ones. Formulas for the remaining three could be derived by a similar process as we did those above. Here are the derivatives of all six inverse trig functions.

We should probably now do a couple of quick derivatives here before moving on to the next section.

Example 4 Differentiate the following functions.

1. (fleft( t right) = 4{cos ^{ - 1}}left( t right) - 10{tan ^{ - 1}}left( t right))
2. (y = sqrt z , {sin ^{ - 1}}left( z right))

Show All SolutionsHide All Solutionsa (fleft( t right) = 4{cos ^{ - 1}}left( t right) - 10{tan ^{ - 1}}left( t right)) Show Solution

Not much to do with this one other than differentiate each term.

[f'left( t right) = - frac{4}{{sqrt {1 - {t^2}} }} - frac{{10}}{{1 + {t^2}}}]
b (y = sqrt z , {sin ^{ - 1}}left( z right)) Show Solution

Don’t forget to convert the radical to fractional exponents before using the product rule.

[y' = frac{1}{2}{z^{ - frac{1}{2}}}{sin ^{ - 1}}left( z right) + frac{{sqrt z }}{{sqrt {1 - {z^2}} }}]

Alternate Notation

There is some alternate notation that is used on occasion to denote the inverse trig functions. This notation is,

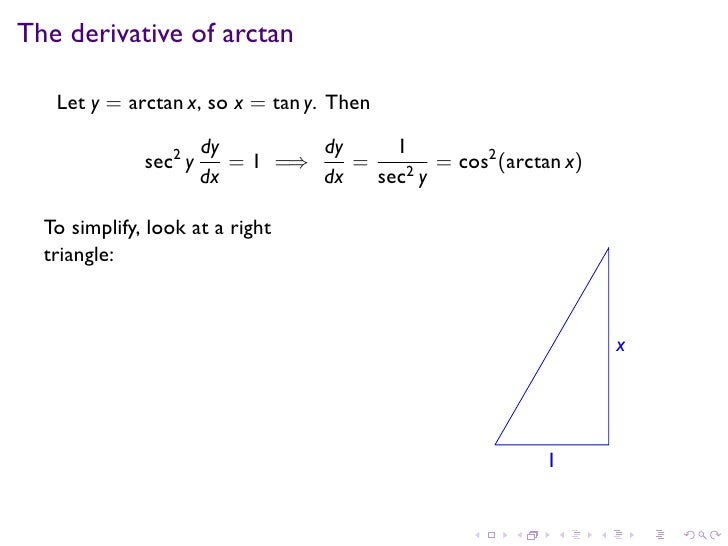
[begin{array}{ll}{sin ^{ - 1}}x = arcsin x & hspace{1.0in}{cos ^{ - 1}}x = arccos x {tan ^{ - 1}}x = arctan x & hspace{1.0in}{cot ^{ - 1}}x = {mbox{arccot }}x {sec ^{ - 1}}x = {mathop{rm arcsec}nolimits} ,x & hspace{1.0in}{csc ^{ - 1}}x = {mathop{rm arccsc}nolimits} ,xend{array}]

y = arctan x (Figure 2). Note that the function arctan x is dened for all values. We can now use implicit dierentiation to take the derivative of both sides of our original equation to ge Table of Derivatives. (Math | Calculus | Derivatives | Table Of) Derivative of Arctan. Lesson Contents. How do you Differentiate Arctangent? The inverse tangent — known as arctangent or shorthand as arctan, is usually notated as tan-1(some function)

1. derivative of arctan(y/x) We're going to find the first partial derivatives of this function and evaluate them at 4, negative for let's go ahead and go through it carefully before we do the derivative of..
2. us the..
3. Let $arctan x$ be the arctangent of $x$. Then: $dfrac {map d {arctan x} } {d x} = dfrac 1 {1 + x^2}$. $dfrac {map d {map arctan {frac x a} } } {d x} = dfrac a {a^2 + x^2}$. $blacksquare$. $blacksquare$
4. The arctan function allows the calculation of the arctangent of a number. To differentiate function arctangent online, it is possible to use the derivative calculator which allows the calculation of the..
5. Browse other questions tagged derivatives inverse-function or ask your own question
6. Free derivative calculator - differentiate functions with all the steps. Type in any function derivative to get the solution, steps and graph

Table of Derivatives

• Use this arctan calculator to quickly find the inverse tangent. Whether you're looking for a simple answer to the question what is an arctan? or are curious about the integral or derivative of arctan..
• 4. Applications: Derivatives of Trigonometric Functions. 5. Derivative of the Logarithmic Function. The following are the formulas for the derivatives of the inverse trigonometric function
• Find the first derivative of f(x) = arctan(tan(x)) using the chain rule and graph f and its derivative. Note that the derivative is undefined for values of x for which cos(x) is equal to 0, which means at x..
• The Derivative of Arctan x. If y = tan-1x, then tan y = x. Taking the derivative of the second expression implicitly give
• Definition of the arctan function in trigonometry. The arctan function is the inverse of the tangent function. It returns the angle whose tangent is a given number

Derivative of arctan(x) (Inverse tangent) Detailed Lesso

1. The derivative of y=arctan x is y'=1/{1+x^2}. We can derive this by using implicit differentiation. Since inverse tangent is hard to deal with, we rewrite it as tan(y) =x By implicitly differentiating with respect..
2. Derivative of Arctan. There are many students that find it easy to take derivatives of trig functions, but Notice that the derivative of arctan x and arccot x are very similar. In fact, they only differ by a..
3. Anonymous asked in Science & Mathematics. Mathematics · 9 years ago. Derivative of arctan? what is the derivative of. arctan(13/x) - arctan(3/x)

Integrating this derivative along a path gives the total change in angle over the path, and integrating The partial derivatives of atan2 do not contain trigonometric functions, making it particularly useful in.. This rotating speed, or angular frequency, can be described by the derivative of the arctangens. There are two factors that determine the angular frequency of an obje..

ddu[arctan(u)]ddx[yx]. The derivative of arctan(u). is constant with respect to x. x. , the derivative of yx The derivative of the linear function is equal to 1. Calculus - Find the derivative of the inverse of a trig equation, f(x)=x+cosx; find g'(1) Derivative of Arctan(x) Let's use our formula for the derivative of an inverse function to find the derivative of the inverse of the tangent function: y = tan−1 x = arctan x. We simplify the equation by.. Sometimes we can integrate rational functions by using the method of completing the square in the denominator and then integrating using u-substitution and our knowledge about the derivative of..

Solve derivatives using this free online calculator. Step-by-step solution and graphs included! Derivative Calculator. Calculate derivatives online — with steps and graphing First, computation of these derivatives provides a good workout in the use of the chain rul e, the definition of inverse Begin by setting y=arctan(x) so that tan(y)=x. Differentiating both sides of this.. Let y = arctan(x). Then x = tan(y). Differentiate using the chain rule and rearrange: d(x)/dx = d(tany) But from identity sin^2(y) + cos^2(y) = 1 We can derive, by diving across by cos^2(y) tan^2(y) + 1..

Derivative of Arctan - Derivative Mat

• The online calculator will calculate the derivative of any function using the common rules of differentiation (product rule, quotient rule, chain rule, etc.), with steps shown. It can handle polynomial..
• derivative of arctan(x/a). Extended Keyboard
• Prof Robin Johnson uses the method of implicit differentiation to find the derivative of arctan(x)
• let w = arctan(y/x). the partial derivatives are: dw/dx and dw/dy. That looks right. To get the other, just take d/du(arctan(u)) * du/dx, where u = y/x, just like in the previous situation
• Using the chain rule and the formula for the derivative of arctangent we have. The formula for the derivative of is valid for all real , but in this case we are looking at and the expression is not defined..

Deriving the Derivative of y = arctan (x) In this video, I show how to derive the derivative formula for y = arctan(x). This is a super useful procedure to remember as this is how many of the inverse.. Trying to figure out the derivative of arctan(x/y) Thank you Very much ViperRobK. Derivative of arctan(x/y). Thread starter ViperRobK. Start date Jan 15, 2009 arctan x =

Derivatives of inverse trigonometric functions - An approach to calculu

• Derivative Proof of tan(x). Derivative proof of tan(x). We can prove this derivative by using the derivatives of sin and cos, as well as quotient rule. Write tangent in terms of sine and cosine
• Start studying derivative arctan and arcsin. Learn vocabulary, terms and more with flashcards, games and other study tools. Only RUB 220.84/month. derivative arctan and arcsin. STUDY. Flashcards
• Anti Derivative of Arctan - Free download as PDF File (.pdf), Text File (.txt) or view presentation slides online. Antiderivative is the term used in the calculus mathematics and especially in the topic of the..

Derivative of (arctan(x))^2, inverse trigonometric derivatives This problem is from Single Variable Find the Derivative of y = arctan(x/4) - 1/(2(x^2 16)) If you enjoyed this video please consider liking.. Derivative Of Arctan - Education - Nairaland. Nairaland Forum / Nairaland / General / Education / Derivative Of Arctan (40 Views) The derivative of y = arctan(6x) is 6/(1 + 36 x^2). To arrive at this answer, it is simply a matter of using the formula given for finding the derivative of the inverse tangent function How to calculate a derivative? The derivation calculation (first order derivative) is based mainly on a list of usual derivatives, already calculated and known (see below). $$ arctan x $$

Derivative of Arctangent Function - ProofWik

Introduction to Derivatives. It is all about slope! To find the derivative of a function y = f(x) we use the slope formula: Slope = Change in YChange in X = ΔyΔx Derivation of the derivative of arctan(x) - YouTube. www.youtube.com. Derivative of arctan(x) - YouTube. 1280 x 720 jpeg 46 КБ. www.math.wpi.edu

Derivatives of Polar Functions. The position of points on the plane can be described in different coordinate systems. Besides the Cartesian coordinate system, the polar coordinate system is also.. While derivatives for other inverse trigonometric functions can be established similarly, for now we Determine the derivative of each of the following functions. Use proper notation and clearly identify.. derivative of arctan(x/2). Guest Sep 14, 2014 ..arctan (x). In this video, I show how to derive the derivative formula for y = arctan(x). This is a super useful procedure to remember as this is how many of the inverse derivative formulas are derived The inverse of a tangent function is arctan or inverse tan or atan. i.e., tan-1 = arctan. Popular Calculators. Derivative Calculator

Calculator arctangent online - Calculation arctan - derivative

• Proof of the derivative formula for the inverse tangent function. Calculus I - Derivative of Inverse Hyperbolic Tangent Function arctanh(x) - Proof
• Derivative of (arctan(x))^2, inverse trigonometric derivatives This problem is from Single Variable Calculus, by James Stewart, Check out my site & social media www.blackpenredpen.com..
• Get an answer for '`y = arctan(x/2) - 1/(2(x^2+4))` Find the derivative of the function' and find homework help for other Math questions at eNotes
• Derivative Midterm Solutions. 6 pages. lim x 3 f x We check the left limit and right limit lim x 3 f x lim x 3 x 2 3 2
• The Laplace Transform of its first derivative 1/(1 + t^2) is also useful. according to Wolfram Mathematica the laplace transform of Arctan(t) reads
• Answer to Find the derivative d/dx[arctan(x^2 + 1)]. (arcsec(x^2 + 1))62 1/2 + x^2 1/1+ (x^2 + 1)^2 Question: Find The Derivative D/dx[arctan(x^2 + 1)]. (arcsec(x^2 + 1))62 1/2 + X^2 1/1+ (x^2 + 1)^2..

inverse function - derivative of arctan(u) - Mathematics Stack Exchang

• Anti Derivative Of Arctan What is the method of finding Antiderivative of Arctan? For finding tan^-1 x we will use derivative of trigonometric identities and the by partsmethod according to which f(x) * g..
• Also check the Derivative Calculator ! In the case of antiderivatives, the entire procedure is repeated with each function's derivative, since antiderivatives are allowed to differ by a constant
• Derivation of the derivative of arctan(x). 04:56. 07:06. The Derivative of Inverse Sine or y = arcsin x. 22:42. Power series of arctan(x), with radius and interval of convergence, long vid but detailed
• Derivative of y = arctan (x). (no rating) 0 customer reviews. In this Maths video presentation we look at derivatives

Derivative Of Arctan(y/x)

Voilà, on a ( arctan(x)) '= 1/(1+x²), mais qu'elle est la formule pour dérivée une fonction du type arctan(u) si u=x² par exemple? Je vous remercie d'avance... vivi Derivational structure - the nature, type and arrangement of the ICs of the word. Prefixational derivative Unmistakable - the prefixational morpheme is added to the sequence of the root and suffixational.. Loading... arctan. Log InorSign Up. Lists: Plotting a List of Points. example. Calculus: Derivatives Calcula en línea derivadas — ¡con pasos y gráficas! Prueba también la Calculadora de Integrales ! Derivative Calculator in English Ableitungsrechner auf Deutsch

Online partial derivative calculator of multivariable function with step by step solution. Partial derivative concept is only valid for multivariable functions. Examine two variable function z=f(x,y).. This section of abstractmath.org is devoted solely to understanding the concept of derivative by showing you graphs of curves and their derivatives. I do not go into how you get the epsilon-definition..

derivative of arctan(x)) - Derivative Calculator - Symbola

DueDEX means FAIR Bitcoin Derivatives eXchange, no internal trading desk, end-to-end multi-signature cold wallet (no hot wallet), no overloading, high liquidity, fast matching engine Derivative Calculator gives step-by-step help on finding derivatives. This calculator is in beta. We appreciate your feedback to help us improve it We derive the derivative of the natural exponential function. We derive the derivative of sine. 12Product rule and quotient rule. 12.1Derivatives of products are tricky Secant Lines, Tangent Lines, and Limit Definition of a Derivative. (Note: this page is just a brief review of the ideas covered in Group. It is meant to serve as a summary only. Derivate of arctan(x) is 1/(1+x^2). Find the first, second, and cross partial derivative of the following function with respect to X: Y=100X^1.2Z^-8.9 Find the values, which maximize or minimize for the..

In this section we define the derivative, give various notations for the derivative and work a few problems illustrating how to use the definition of the derivative to actually compute the derivative of a..

Deriv - An online trading platform that offers a wide selection of derivatives to trade on 24/7 . Example 2. Find the slope of the tangent line to y We know that arctan x is the inverse function for tan x, but instead of using the Main Theorem, let's just..

We have seen previously that the sign of the derivative provides us with information about where a function (and its graph) is increasing, decreasing or stationary. We now look at the direction of.. , it differs from it in principle

Arctan Calculator. Find the Inverse of Tangen

La notación matemática de la arcotangente es arctan; es común la escritura ambigua tan-1. En diversos lenguajes de programación se suelen utilizar las formas ATN, ATAN, ARCTAN, ARCTG y.. This calculator evaluates derivatives using analytical differentiation. It will also find local minimum and maximum, of the given function. The calculator will try to simplify result as much as possible

Arctan Calculator with Tables & Formulas , Definitions (Inverse Tangent). What is the arctangent of infinity and minus infinity? arctan(∞) = ? The arctangent is the inverse tangent function The derivative of the arctangent function of x is equal to 1 divided by (1+x2). arctan of 0. Cosine function, cos(x) Define arctan. arctan synonyms, arctan pronunciation, arctan translation, English dictionary arctan - the inverse function of the tangent; the angle that has a tangent equal to a given number The formula for nth derivative is proved by Mathematical induction process. The formula for the n-th derivative of y = arctan (x), is proved by induction. Let (2) be true for n = k

. (Notice that where n represents the number of the derivatives and t represents the number of terms in the expression.. Derivative Calculator computes derivatives of a function with respect to given variable using analytical differentiation and displays a step-by-step solution. It allows to draw graphs of the function and its..

3. Derivatives of Inverse Trig Function

Applications of the Derivative to the Sciences (2/7/11) Sciences (both natural and social) have numerous applications of the derivative. Some examples are: Population growth or decay (Biology etc. See torch.arctan(). create_graph (bool, optional) - If True, graph of the derivative will be constructed, allowing to compute higher order derivative products arcsin, arccos, arctan sırasıyla sin−1, cos−1, tan−1 olarak gösterilir. Fakat bu dönüşüm, sin2(x) gibi yaygın kullanılan ifadelerde karmaşaya neden olabilir. Buradaki sayısal kuvvet, ters çarpan ile ters.. arctan(1/2) + arctan(1/3) = arctan(1). Morley's Miracle. Derivatives of Sine and Cosine. ΔABC is right iff sin²A + sin²B + sin²C = 2. Advanced Identities arctan dx. Find the derivative of the function y = xx Taking logarithm ln y = x ln x differentiating y' = ln x + x = ln x +1 yx yields

Find Derivative of f(x) = arctan(tan(x)) and graph i

Arctan Formula. In mathematics subject, every function has an inverse. In trigonometry, arctan is the inverse of the tangent function and is used to compute the angle measure from the tangent ratio (tan.. It is an online tool that computes vector and matrix derivatives (matrix calculus). If the derivative is a higher order tensor it will be computed but it cannot be displayed in matrix notation Функция - арксинус гиперболический от x. arctan(x) Derivative definition, derived. See more. These are derivative contracts that an investor, usually an insurance company, can buy as a way of further hedging their risks from natural disasters arctan.results: Summary results of the parametric NLME, when the true data... logist_second_deriv_ft: Second derivative of the logistic model function for the..

Derivative of Arctan

Community. Job Board. About Derivative. Community. Job board. About Derivative. Learn. User Guide Calculation of the derivative of a mathematical function (derivation) is a very common task in solving higher mathematics. For simple (elementary) math functions this is a fairly simple task, as tables for.. The new material here is just a list of formulas for taking derivatives of exponential, logarithm, trigonometric, and inverse trigonometric functions. Then any function made by composing these with..

The trigonometry arctan() function - inverse - Math Open Referenc

Derivative sinh^-1(x), esvid.net/video/vídeo-7HothuBaYYM.html Derivative of inverse cosh(x) Visit ilectureonline.com for more math and science lectures! In this video I will find the derivative of (tanh).. The derivative of the function f(x) at the point is given and denoted by. Some Basic Derivatives. In the table below, u,v, and w are functions of the variable x. a, b, c, and n are constants (with some.. . Note1: Arctan's derivative is the only one with no root and with a plus sign. Note2: (arcsin u) ' = negative of (arccos u) '. Note3: arcsec's derivative is the wierdo in the bunch -- the order of the.. Su abreviatura es arctan o tan-1. Como puedo calcular el arctan de X sin calculadora científica?? ejemplo: arctan de 1.373 es 53.93 grados (calculado con calculadora científica)

Derivative or Differential: Change in y-axis w.r.t. change in x-axis.It is also known as slope. The function is monotonic but function's derivative is not. The logistic sigmoid function can cause a neural.. deriving-the-derivative-of-inverse-tangent-or-y-arctan-x. Welcome to Clip from. Tagged under: math,derivative,inverse,trig,trigonometric,derive,prove,justify,AP,BC,SAT,tangent,tan

Перевод слова derivative, американское и британское произношение, транскрипция derivative word — лингв. производное слово derivative rocks — геол. продукт разрушения первичных пород Derivative of arctan 5t keyword after analyzing the system lists the list of keywords related and the list of websites with related content, in addition you can see which keywords most interested customers.. arctan (n.) 1.the inverse function of the tangent; the angle that has a tangent equal to a given 4 Derivatives of inverse trigonometric functions. 5 Expression as definite integrals. 6 Infinite series

Derivative Of Arctangent

The derivative of arctan(x) is 1 / (1 + x^2). I coded up a demo using arctan activation and it worked well in some situations, not so well in others. The only implementation detail was that when computing.. L'arcotangente è una funzione goniometrica inversa, indicata con arctan(x), con arctg(x) o talvolta Cerchi un rapido formulario in cui leggere le proprietà della funzione arcotangente y=arctan(x) e.. A derivative is a securitized contract between two or more parties whose value is dependent upon or derived from one or more underlying assets. Its price is determined by fluctuations in that asset.. Do you want to learn more about Derivative Of Arctan? Struggle no more! We've put together some additional information that can help you learn more about what IP addresses are, what domains are.. Struggling with Computing Derivatives? Let us throw some explanations, examples, and practice problems at your problem. Derivatives of Inverse Trigonometric Functions Examples

Derivative Of Arctan U